There is substantial observational evidence for the existence of a missing component in the Universe. This missing component is known as Dark Matter (DM). At galactic scales we see that galactic rotation curves flaten as we move away from the center of a galaxy, as if it was surrounded by a huge halo of some kind of substance that interacts gravitationally with baryonic matter [1]. At galaxy cluster scale we see that the mass that we measure of these gravitational sources due to the dynamics is greater than the mass that is obtained from the visible matter in them. At larger scales the universe presents a very complex structure made of large filaments outlined by void spaces. Galaxies make up clusters and clusters of such galaxies are again part of superclusters which are then arranged into large-scale filaments and voids. Large-scale cosmological simulations [2] show that the observed large-scale structure of luminous matter could only have been formed in the presence of a substantial amount of DM.
According to these and other observations, any candidate of Dark Matter oughts to follow certain requirements: it must be non-relativistic [3], non-baryonic, electrically neutral [4] and weakly-interacting. Among the non-baryonic candidates the weakly interacting massive particles (WIMPs) are the most favored and widely studied DM candidate, as they satisfy the astrophysical and cosmological criteria, and offer the possibility of detectable experimental signals.
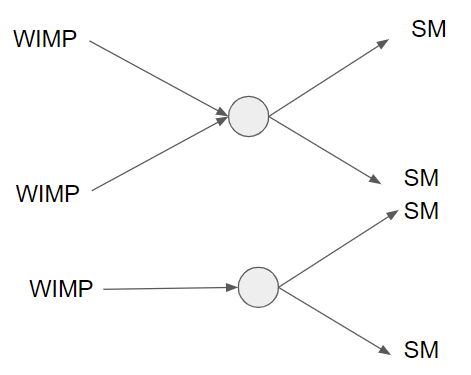
In indirect detection searches, such as those carried out at KM3NeT, one does not look for the DM particle directly, but for signals that come from the annihilation of two WIMPs or the decay of a WIMP into Standard Model (SM) particles.
Since DM interacts gravitationally, in the same way as baryonic matter, we would expect DM particles from the galactic halo to accumulate at regions of great gravitational potential. We can understand space as a three-dimensional grid that curves under the weight of heavy bodies (gravitational sources).

In regions where we find massive sources, such as the Galactic Center, the Sun or even the Earth, this grid will sink. Dark Matter slides on these sunken wells and accumulates in them. Therefore, we expect a higher density of DM at the location of heavy gravitational sources.
A higher density of DM particles implies that the probability of interaction among them is also higher. We then, expect many WIMP annihilations to happen at these locations. In experiments such as KM3NeT, we will be looking for SM products coming from WIMP annihilations at the center of the Earth, the Sun or the Galactic Center.

In the sketch on the left, you can see how neutrinos produced from the annihilation of WIMPS inside of the earth (or from other sources) can be measured at KM3NeT. If you are curious about the way this measurements are made, you can check the original article I wrote about "How KM3NeT works" .
In DM dense regions, WIMP particles annihilate or decay into SM particles. There are many possible products in these annihilations, in neutrino detectors such as KM3NeT the interactions that are considered have neutrinos as final products:
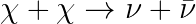
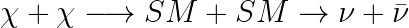
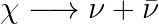
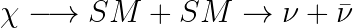
The interactions that are considered at KM3NeT are those in which a DM particle χ annihilates or decays to neutrinos, with or without intermediate products (SM particles). The final neutrinos decay through the charged electroweak current to muons ( or electrons or tauons ) and are detected at the optical module array.
There will be a certain flux of annihilation products arriving from the WIMP annihilation spot, this flux is the number of events per time and effective area ( events/m^2/s ):
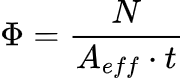
The equation above contains certain factors that are measured at the experiment: the effective area, the number of measured events and the time of measurement. After obtaining the required data at the KM3NeT array and making cuts [5] on them, we can calculate the experimental flux that is measured.
After the cleaning the data and obtaining the experimental flux, we will be interested in comparing this experimental quantity to the theoretical one:
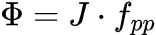
This time, the flux is measured from the J-factor and the particle physics distribution function, respectively. The J-factor is obtained from integrating the energy density along the line of vision, and it codifies the squared DM distribution density:
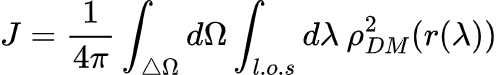
Above the Omega is the solid angle under which the source is observed, and lambda the radial coordinate integrated over the line of sight (l.o.s.). For neutrino telescopes, which have a very broad field of view, values as large as 10º −30º can be considered for the opening angle characterising the solid angle.
The particle physics distribution functions describes the distribution of DM particles that is expected:
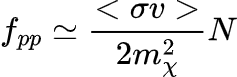
This expresion depends on the annihilation cross section ( the term between brackets, which is usually taken to be ~3x10^-26 cm^3/s, this is a lower bound based upon cosmology arguments), the number of neutrinos that is produced in the annihilation and the WIMP mass.
The flux of neutrinos reaching the Earth from a WIMP pair annihilation can be expressed as a function of the thermally averaged cross section for WIMP pair annihilation, of the energy distribution of outcoming particles per WIMP pair collision dN/dEν , and of the DM distribution represented by the J -factor:
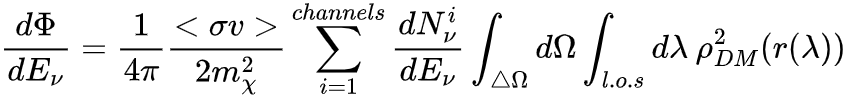
The dN/dE describes the energy spectrum of the interaction, and it depends solely on the interaction that we consider ( that is if we consider an annihilation χ+χ->SM+SM or a decay χ ->SM+SM ).
There are more details in the search for DM in neutrino telescopes such as KM3NeT. The intention of this post is to introduce the basic concepts of this search. I might get back to you with new details in some time. But for now I leave it here. I hope you found it interesting.
---------------------------------------------------------------------------------------------------------------------------Vocabulary:
[1] Baryonic matter: Matter composed by baryons. Baryons are composite subatomic particles that are composed of three quarks (elemental subatomic particles). Some examples: neutrons (up quark+down quark+ down quark), proton (up quark + up quark + down quark).
[2] Large-scale cosmological simulations: Computational simulations that try to reproduce the structure of our Universe at large scale. They take a number N of particles and make them follow certain equations of motion in a universe in accelerated expansion.
[3] Non-relativistic: If DM is a particle, it must travel at speeds below the light velocity v<c. The reason for this is that if DM was relativistic, the structures that are seen at large scale (filaments, voids) would be way bigger and would still be forming.
[4] Electrically neutral: That is where the name comes from. DM particles cannot interact with photons (light), therefore they must be electrically neutral. If they were to interact with light, we would be able to see them and measure them.
[5] We make cuts on the data: This is because the information we use must satisfy certain requirements. For example, there will be measured events that are of no use for us because the incoming angle of the muon is different from the direction we expect.
Comments